Modelling the propagation of infectious disease via transportation
An additional row must be included on outlet the transportation matrix
Share. Visit »
Transportation Problem Set 3 Least Cost Cell Method
Land Free Full Text The Flow Matrix Offers a Straightforward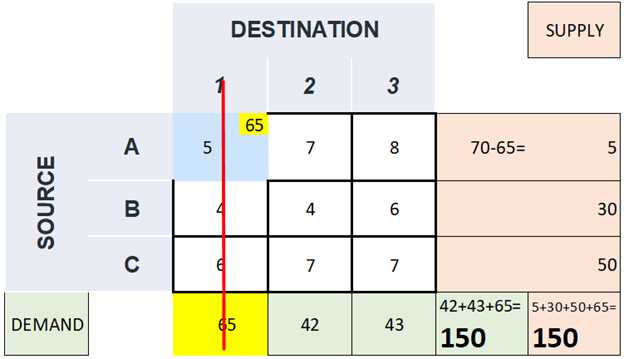
optimize Transportation problem Towards Data Science
Overview of the adjacency matrix based contraction sequence search
Logistics Free Full Text International Transportation Mode
1 Flow chart for Assignment problem 3.6Hungarian Method Algorithm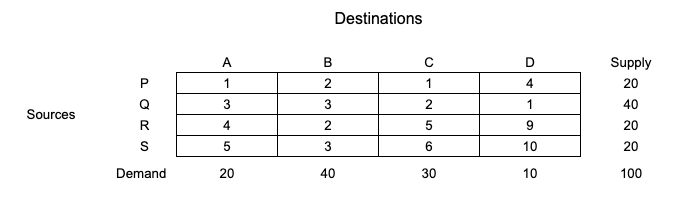
Tie in selecting row and column Vogel s Approximation Method
J. Intell. Free Full Text Should Intelligence Tests Be Speeded
Solved Q3 B Mark the letter of the correct answer Chegg
Shimbel Distance Matrix D Matrix The Geography of Transport
Solved Need to add extra column in existing Power BI matr
Module 4 Transportation Problem and Assignment problem
Asssignment problem PDF
SE LESSON 3. TRANSPORTATION PROBLEMS
Solved Need to add extra column in existing Power BI matr
Valued Graph Matrix L Matrix The Geography of Transport Systems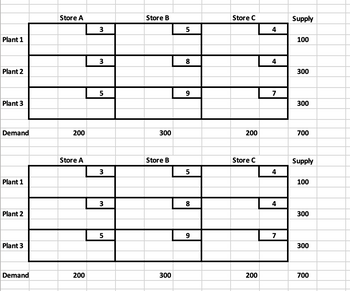
Answered Using the transportation matrices bartleby
Numerical on assign.pdf ASSIGNMENT MODEL Assignment model is an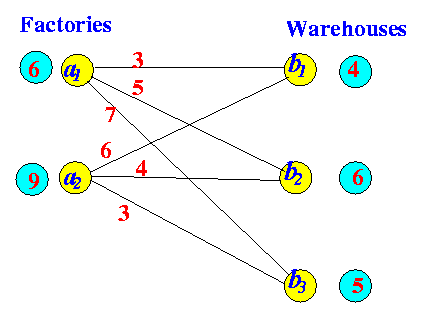
The Hungarian Algorithm for the Transportation Problem
Vogel s Approximation Method VAM Steps Solved Example
Transportation Problem Set 2 NorthWest Corner Method
3rd EDITION STATE l UNIVERSITY ppt video online download
Module 4 Transportation Problem and Assignment problem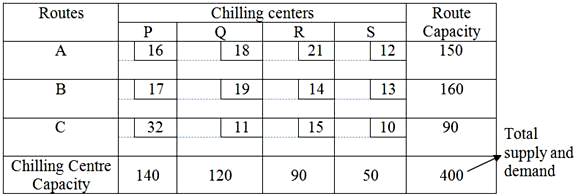
image001.jpg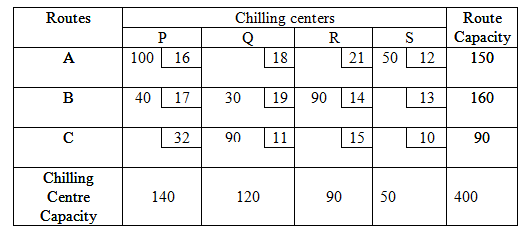
image012.png
Module 4 Transportation Problem and Assignment problem
Assignment Model Operation Research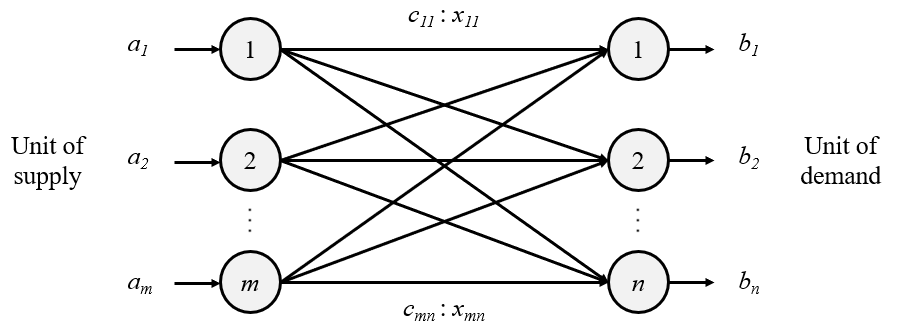
Operations Research with R Transportation Problem by Roberto
Transportation Problem Set 3 Least Cost Cell Method
Tutorial 8 1. For a balanced transportation problem Chegg
MODI Method Operations Research PPT
Balanced and Unbalanced Transportation Problems Definition
Transpose Wikipedia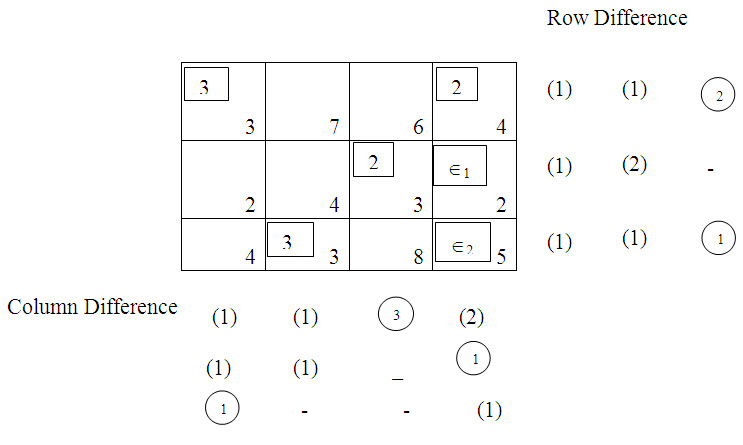
SE LESSON 3. TRANSPORTATION PROBLEMS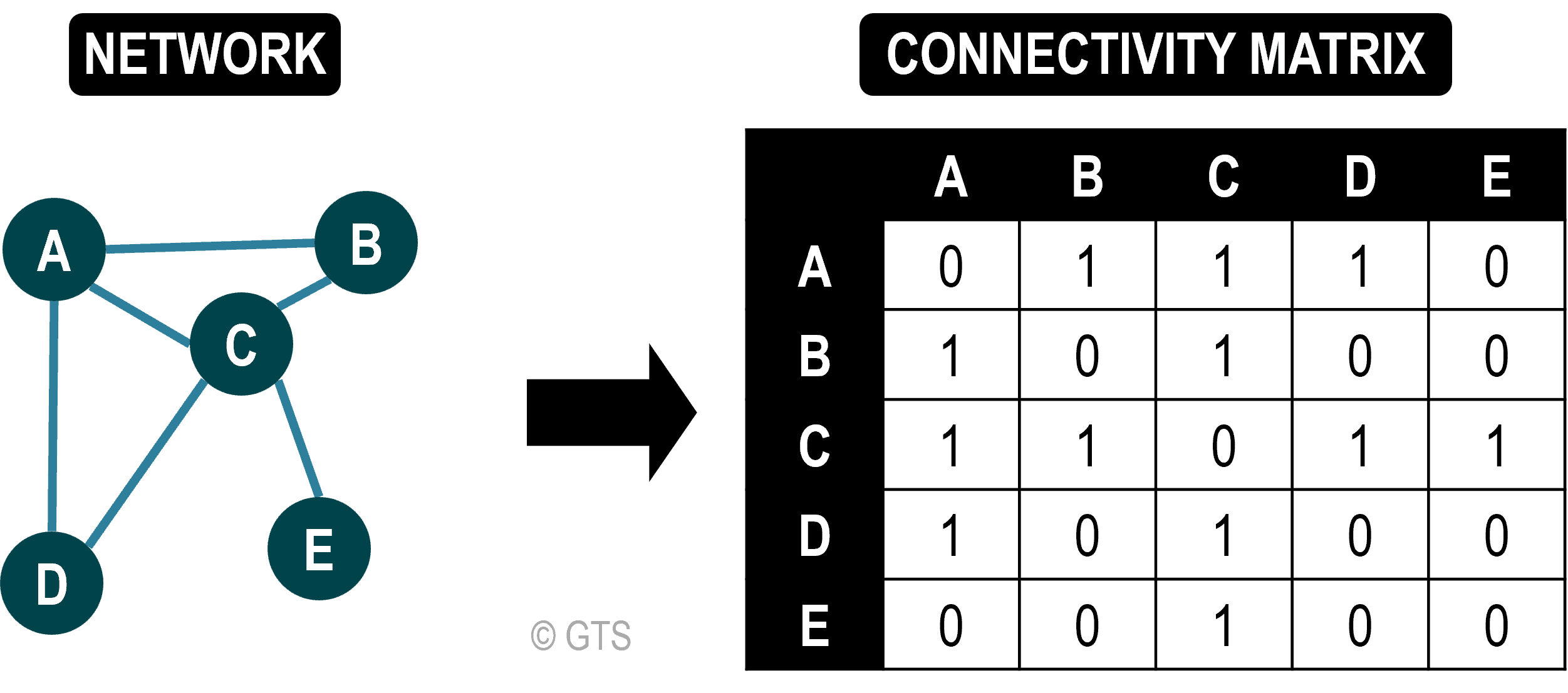
Simple Connectivity Matrix The Geography of Transport Systems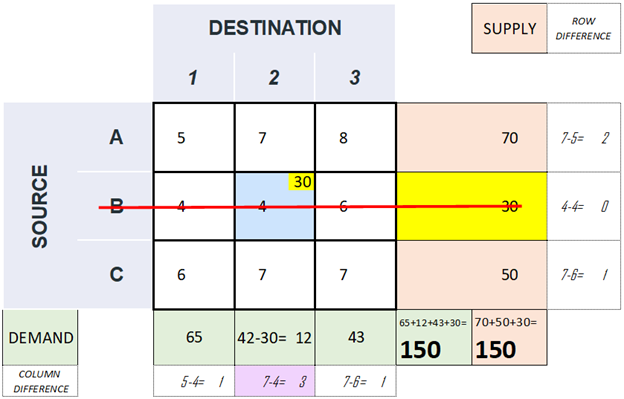
optimize Transportation problem Towards Data Science
Sparse Matrix Limitations Best Practices BotPenguin
image008.png
Transportation problem example. The user supplies signs and values